Grade 10 Circles @1

CIRCLES 

1. The radius of a circle is 8 cm. Calculate the length of a tangent drawn to this circle from a point at a distance of 10 cm from its centre?

2.In the given figure, O is the centre of the circle and AB is a tangent to the circle at B. If AB = 15 cm and AC = 7.5 cm, calculate the radius of the circle.

3.

Two circles of radii 5 cm and 3 cm are concentric. Calculate the length of a chord of the outer circle which touches the inner.

4.From the given figure prove that:

AP + BQ + CR = BP + CQ + AR.

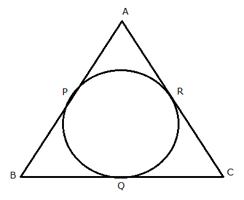
Also, show that AP + BQ + CR = x perimeter of triangle ABC.

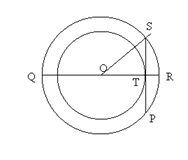
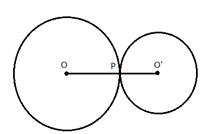
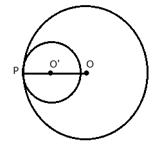
5.Radii of two circles are 6.3 cm and 3.6 cm. State the distance between their centers if -

i) they touch each other externally.

ii) they touch each other internally.

6.In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that:

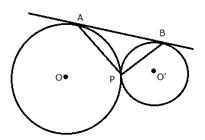
i) tangent at point P bisects AB.

ii) Angle APB = 90°

7.Two parallel tangents of a circle meet a third tangent at point P and Q. Prove that PQ subtends a right angle at the centre.

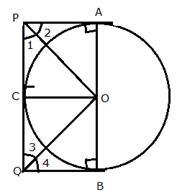
8. ABC is a right angled triangle with AB = 12 cm and AC = 13 cm. A circle, with centre O, has been inscribed inside the triangle.

Calculate the value of x, the radius of the inscribed circle.

9.

In a triangle ABC, the incircle (centre O) touches BC, CA and AB at points P, Q and R respectively. Calculate:

i) 

ii) 

given that 

10. In the following figure, PQ and PR are tangents to the circle, with centre O. If , calculate:

i) 

ii) 

iii)

ANSWERS